本次加拿大作业案例分享是一个数学代写的练习题
[3] 1. Let C be the curve given by the intersection of the cylinder x2 + z2 = 9 and the plane z = 2x ! y.Assume counter-clockwise orientation when seen from the positive y-axis.
(a) Find a parameterization of C.
(b) Describe, in words, what it means for C to be a field line of a vector field F .
[6] 2. Let F (x, y) = (x2y2, y4) and let C be the positively-oriented rectangle with vertices (0, 0), (2, 0),(2, 1), and (0, 1).
(a) Find RC F · dx by setting up and evaluating the appropriate definite integral(s).
(b) Find RC F · dx using Green’s Theorem. Confirm that you get the same result as in (a).
3. Let
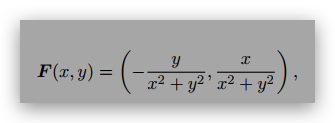
and let C be the positively-oriented diamond with vertices (1, 0), (0, 1), (!1, 0), and (0, !2). Find
RCF · dx. Hint: choose your technique wisely.
4. Let ⌃ be the part of the cone z = px2 + y2 inside the cylinder x2 + y2 = 9. Assume the normal points inward (towards the z-axis).
(a) Find a parameterization of ⌃.
(b) Let F (x, y, z) = (x, y, 0). Find the surface integral of F on ⌃.
5. Consider the vector field F (x, y, z) = (x2 + y2, 0, z).
(a) Find the divergence r · F .
(b) Write F in cylindrical coordinates.
(c) Now, find r · F in cylindrical coordinates.
(d) Confirm that your results in (a) and (c) are the same.
6. Use Cartesian Tensor notation to prove that a ⇥ (b ⇥ c) = (a · c)b ! (a · b)c, where a, b, and c are vectors.
EasyDue™ 支持PayPal, AliPay, WechatPay, Taobao等各种付款方式!
E-mail: easydue@outlook.com 微信:easydue
EasyDue™是一个服务全球中国留学生的专业代写公司
专注提供稳定可靠的北美、澳洲、英国代写服务
专注提供CS、统计、金融、经济、数学等覆盖100+专业的作业代写服务