本次加拿大代写作业案例分享是一个证明题的project数学代写
In this mini-project, we will investigate sine and cosine series of the function f(x) = π/2−x/2 on 0 ≤ x ≤ π.
1. Let’s start by considering the even extension of f(x). Find the cosine series of f(x).
2. To what function does the cosine series in (1) converge pointwise on [−π; π]? State the function and sketch it on [−π; π].
3. Use the Weierstrass M-Test to prove that the cosine series in (1) converges to the function in (2) uniformly. (You must use the M-Test, not the Theorem on Uniform Convergence of Fourier Series.)
4. Now, let’s consider the odd extension of f(x). Find the sine series of f(x).
5. What is an important difference between the an’s in (1) and the bn’s in (4) that may affect conver gence?
6. To what function does the sine series in (4) converge pointwise on [−π; π]? State the function and sketch it on [−π; π].
7. The sine series exhibits the Gibbs Phenomenon. Let’s investigate the overshoot near x = 0. Let SN(x) be the partial sum of the first N terms in the sine series. Show that
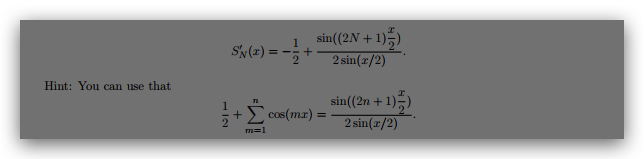
8. (Bonus) Show that the first local maximum of SN(x) with x > 0 occurs at x( max N) = π/N + 1.
9. Calculate SN(x( max N) ) at different values of N to investigate what happens as N increases. Discuss whether your results seem consistent with the claim made in lecture 30, slide 5, that the overshoot amplitude is approximately 9% of the size of the jump discontinuity. (Use can use a computational tool of your choice for this: Matlab, Maple, Octave, Excel, Python, programmable calculator, etc.)